ÃÈÃÃÉçÇø

In a new report on Science Advances, Chen Fang and Liang Fu from the Beijing National Laboratory for Condensed Matter ÃÈÃÃÉçÇøics in China, Kavli Institute for Theoretical Sciences and the Department of ÃÈÃÃÉçÇøics, Massachusetts Institute of Technology in the U.S. Detailed the discovery of new types of quantum anomalies in two-dimensional systems with time-reversal symmetry (T) (conservation of entropy) and discrete ; where a shape retains the same structure after rotation by a partial turn and order. They then physically realized anomalous states on the surface of new classes of (TCIs) normal to the rotation axis and supporting a . The presence of helical modes allowed them to form a new quantum device from a topological crystalline insulator known as a helical nanorod with quantized longitudinal conductance.

A single flavor of (elementary particles) can have quantum anomalies where the conservation of is . Well-known examples include the chiral anomaly of Weyl fermions in (3-D), and parity anomaly . In the present work, Fang and Fu presented a new quantum anomaly associated with (T) and discrete rotational symmetry (C_n = 2, 4, 6). Such anomalies could only exist in theories that broke continuous rotation symmetries in 2-D. Specific materials such as TCIs (topological crystalline insulators) can host robust surface states that have a dispersion relative to massless carriers. Breaking the protective symmetry within such materials can cause the carriers to acquire mass.

Forming new classes of TCIs

In TCIs, topology and crystal symmetry intertwine to form surface states with distinct characteristics. Breaking crystal symmetry in TCIs can impart mass to massless Dirac fermions; therefore, the presence of topological surface states protected by crystal symmetries is a defining property of TCIs. The that describe all possible crystal symmetries allow for many different classes of TCIs. Researchers had previously found a class of TCI protected by reflection symmetry and another class of TCI protected jointly by glide reflection and time-reversal symmetry within to form experimental , while theorizing of TCIs.

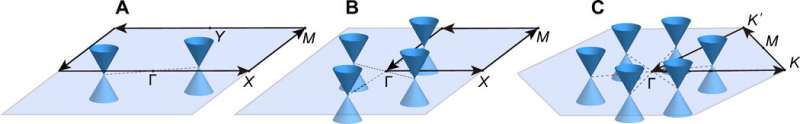
The to find topological materials involves computing the band structure of a particular material to understand the electronic states, then feed this information to a formula to reveal if the material is topological. In addition, the electronic band structure, known as the relationship between the energy of an electron and its quasi-momentum, can determine if a material is a metal or an insulator. Researchers had to predict and experimentally discover such topological materials. In the present work, therefore, Fang et al. predicted a new class of TCIs jointly protected by n-fold rotation and time-reversal symmetry to exhibit topological surface states containing massless Dirac cones on the top and bottom surfaces.

Understanding rotational anomaly

The study of anomaly led them to theorize new classes of (where the underlying laws are not sensitive to the direction of time) TCIs with C_n = 2,4,6 rotation symmetry. These TCIs had anomalous surface states on the top and bottom surfaces. For each new class of TCIs, the team constructed the corresponding topological invariant relative to in momentum space. Based on (number of random variables or attributes under consideration) and (magnetic structures of finite width that separate regions of uniform magnetization within a magnetic material), the scientists further provided a unified real-space understanding of these TCIs. They predicted several materials to realize the anomalous surface states protected by two- and four-fold rotation symmetries. The researchers then proposed a new quantum device based on the anomalies, known as the "helical rod," made using these new TCIs.

Topology in momentum space and topology in real space

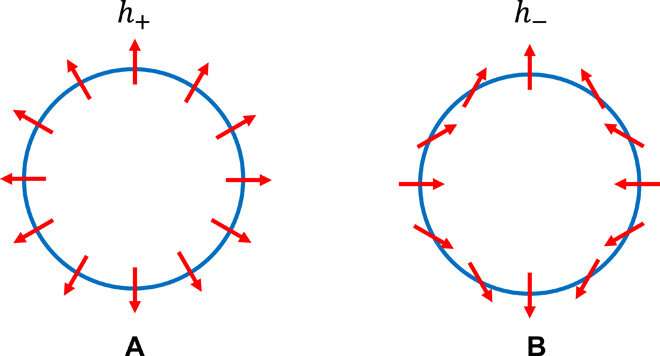
To construct the new TCIs and form topology in momentum space, Fang et al. could add two time-reversal invariant (T-symmetry), strong topological insulators (TIs) each with n-fold rotational symmetry. They considered the symmetry-allowed hybridization between the surface Dirac fermions to TIs to obtain the desired surface states of TCIs. The researchers described the Dirac fermions using h₊ and h_- vortex-like spin textures in momentum space with left and right-handed chirality. The researchers observed the presence and absence of continuous rotation symmetry by looking at the pseudospin vector pattern on some equal energy contours of h₊ and h_- respectively.

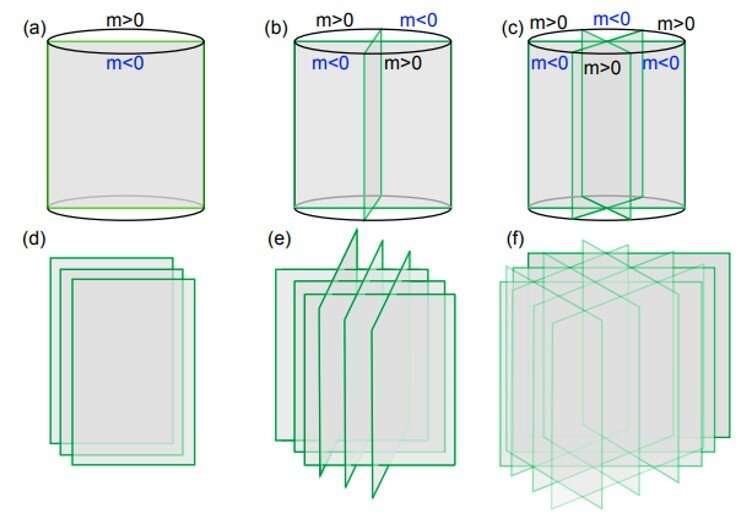
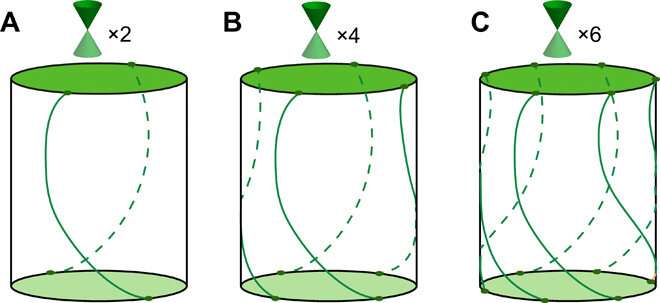
After establishing the TI surface state band structure, Fang et al. provided an alternative explanation of their topological nature from the , similar to a previous research approach. The real-space approach added symmetry-allowed perturbations to break translational symmetry and gap the massless Dirac fermions on the surface for further study. The phenomena facilitated nontrivial TCI states and demonstrations of their robustness under electron interactions. For this, they considered a double-TI model of a TCI placed within a cylinder of a size greater than the correlation length and surface, smooth at the atomic scale. For C_4,6-TCI placed on a cylinder, locations of the modes on the surface states were not pinned to any physical hinges or . Although the cylindrical shape contained continuous rotation symmetry, the system described in the study broke it down to discrete rotational symmetry, to indicate the existence of 1-D gapless lines even on a perfectly atomic-scale smooth cylinder.

Fang et al. then noted the possibility of understanding topological crystalline states from a dimensional reduction perspective, where the 3-D state could be considered as a set of decoupled layers of 2-D topological states. All three types of new TCIs introduced in this work could therefore be constructed from 2-D TIs. Fang et al. used this construction to extend their theory of strongly interacting symmetry-protected topological states protected by rotation symmetry and any local symmetry including, but not limited to time-reversal symmetry.

Since 1-D helical modes are well known to be free from back-scatter due to time-reversal symmetry, this unique property allowed Fang et al. to design a helical nanorod from these new materials. Each helical mode only required time-reversal symmetry for protection and the rotation symmetry ensured that the n-helical modes did not cross each other in real space and gap out. In this way, as long as the rotation symmetry was not considerably broken, these helical edge modes would remain stable, although they no longer related to each other via a rotation—to form new classes of TCIs with surface rotation anomaly.

© 2020 Science X Network